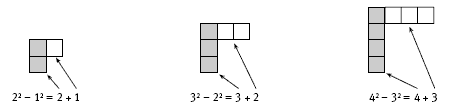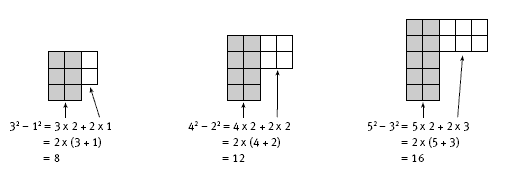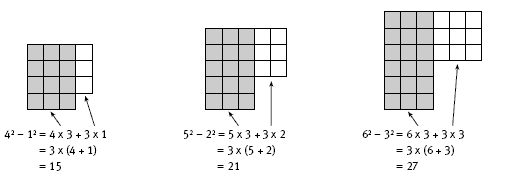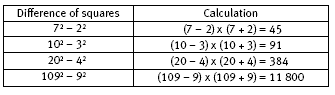This is a level 5 algebra strand activity from the Figure It Out series.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (246 KB)
find a pattern in square number sequences
use a pattern to make a prediction
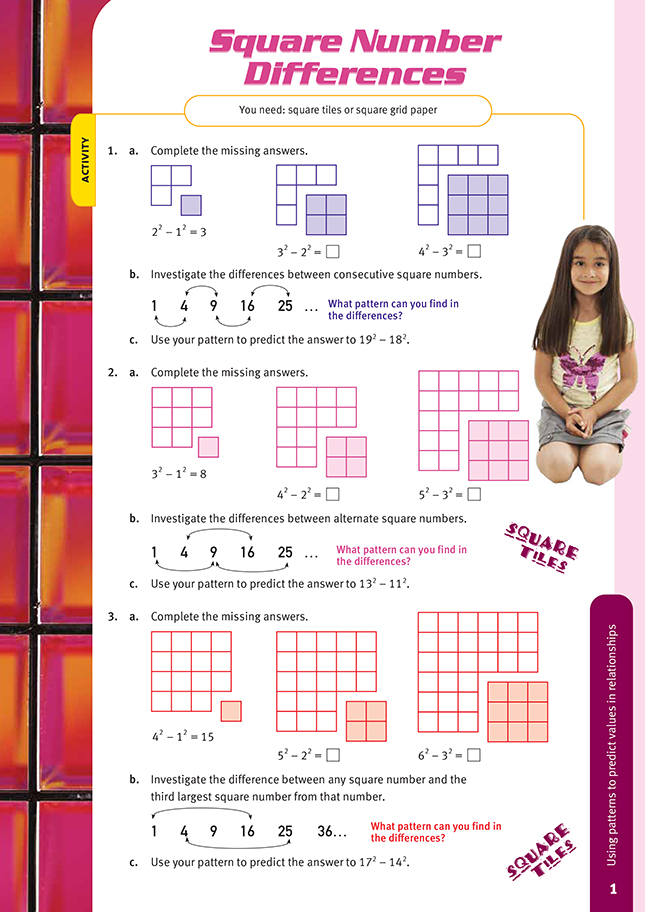
FIO, Level 4+, Algebra, Book Four, Square Number Differences, page 1
square tiles or square grid paper
In this activity, students look for patterns in square tile arrangements. Showing differences with tiles, multilink cubes, or drawings on squared paper is likely to help the students see the various patterns.
In question 1, the tile arrangements relate to the differences between consecutive square numbers. When 32, represented by a 3 by 3 square, is compared with 22 (a 2 by 2 square), the difference is 5 squares, represented as an L-shaped design.
The differences between two consecutive square numbers, for example, 22 – 12, 32 – 22, or 42 – 32, can be shown as follows:
By considering the equations or the diagrams, the students may see a simple rule for these calculations, in which the difference between the numbers being squared is 1. The rule is: add the two numbers that are being squared. For example, 72 – 62 can be represented as 7 + 6 = 13, and 192 – 182 as 19 + 18 = 37. This
rule can also be expressed algebraically as: “If b = a + 1 (where a and b are consecutive numbers), then b2 – a2 = a + b.” The diagrams above should help the students to understand geometrically why the rule works. For example, 202 – 192 = 20 + 19 (which is b2 – a2 = a + b for the values a = 19, b = 20)
= 39
In question 2, the pattern for the differences between alternate square numbers can be shown as follows:
The students may see a simple rule for these calculations, in which the difference between the numbers that are squared is 2. The rule is: double the sum of the two numbers that are squared. So, for example, 272 – 252 = 2 x (27 + 25).
Once again, the diagrams above will help the students to understand geometrically why the rule works.
Algebraically, the rule here is: if b = a + 2, then b2 – a2 = 2(a + b).
In question 3, the pattern for the difference between any square number and the third following square number can be shown as follows:
The students may see a simple rule for these calculations, in which the difference between the numbers that are squared is 3. The rule is: treble the sum of the two numbers that are squared. So, for example, 272 – 242 = 3 x (27 + 24). Algebraically, the rule here is: if b = a + 3, then b2 – a2 = 3(a + b).
These rules can be further generalised. The following tables summarise the calculations for questions 1 to 3. For each of these tables, the short cut calculation is always the difference multiplied by the sum of the two numbers that are squared. Note that in the first table below, the difference is 1, so we can write 4 + 3 instead of 1 x (4 + 3), and so on.
In general, then, the rule is: b squared minus a squared equals the difference between b and a multiplied by the sum of b and a. Algebraically, we can write this as:
b2 – a2 = (b – a) x (b + a)
= (b – a)(b + a).
This rule will work for all values of a and b. The following table shows how this rule can be used:
Answers to activity
1. a. 32 – 22 = 5 and 42 – 32 = 7
b. The difference between consecutive square numbers is always odd. The difference is the sum of the two numbers that are squared.
c. 19 + 18 = 37, so 192 – 182 = 37
2. a. 42 – 22 = 12 and 52 – 32 = 16
b. The difference between alternate square numbers is always even; it is twice the sum of the two numbers that are squared.
c. 2 x (13 + 11) = 48, so 132 – 112 = 48
3. a. 52 – 22 = 21 and 62 – 32 = 27
b. The difference is always odd; it can be worked out by trebling (multiplying by 3) the sum of the two numbers that are squared.
c. 3 x (17 + 14) = 93, so 172 – 142 = 93